How to Convert Degrees to Radians in Pi
What is the deal with radians anyway?
Most of you are used to thinking of a circle in terms of degrees: 360° is the whole circle. 180° is half the circle etc... Well, radian measure is just a different way of talking about the circle. Radian measure is just different unit of measure.
Just as we can measure a football field in yards or feet -- we can measure a circle in degrees (like the good old days) or in radians (welcome to the big leagues!)
Think about what the word radian sounds like... well, it sounds like 'radius', right? It turns out that a radian has a close relationship to the radius of a circle.
So what is a radian then?
Definition of radian:
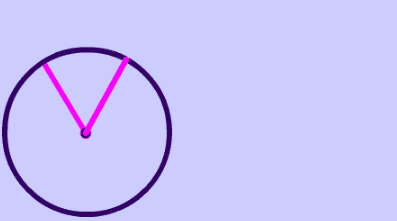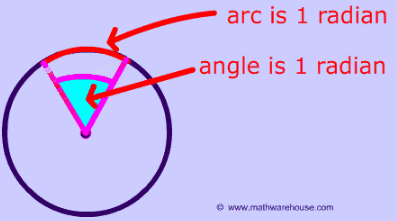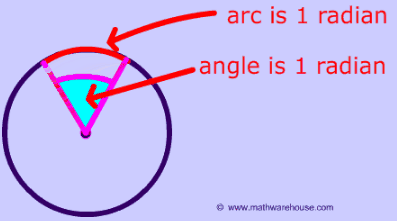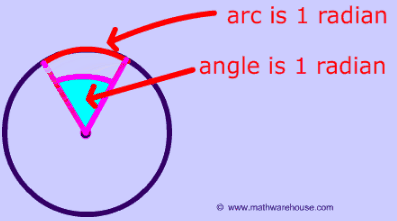
a radian is the measure of an angle that, when drawn as a central angle of a circle, intercepts an arc whose length is equal to the length of the radius of the circle.
This definition is much easier understood by looking at the demonstration immediately below.
Demonstration of Radian of Circle
The gif below is an animation of 1 radian. Notice how the length of 1 radius stretches out to a portion of the circle. That portion is 1 radian of the circle.
There is a simple formula to convert radians to degrees. $$ 1 \pi \text{ radian } = 180^{\circ}$$ . Therefore you can easily convert from one unit of measure to the other.
Degrees Radians
The general formula for converting from degrees to radians is to simply multiply the number of degrees by $$ \red { \frac {\pi}{ 180^{\circ}} }$$ .
Example 1
Convert 200° into radian measure:
$ 200 ^{\circ} \cdot \red { \frac {\pi}{ 180^{\circ}} } \\ = \frac{10\pi}{ 9} \text{ radians } \\ \approx \text{3.49 radians } $
Example 2
Convert 120° into radian measure:
$ 120 ^{\circ} \cdot \red { \frac {\pi}{ 180^{\circ}} } \\ = \frac{2\pi}{ 3}\text{ radians } \\ \approx \text{2.09 radians } $
Radians Degrees
The general formula for converting from radians to degrees to simply multiply the number of degree by $$ \red{\frac{180^{\circ}}{\pi} } $$ .
Example 1
Convert $$ \frac{4}{9} \pi \text{ radians} $$ to degrees.
$ \frac{4\pi}{9} \cdot \red{\frac{180^{\circ}}{\pi} } \\ = \frac{4\pi \cdot 180 ^{\circ} \cdot}{9\pi} \\ =\frac{720 ^{\circ} \pi \cdot}{9\pi} \\ \frac{720 ^{\circ} \cancel{ \color{Red} \pi} \cdot}{9\cancel{ \color{Red} \pi}} \\ = 80 ^{\circ} $
Example 2
Convert 1.4 radians into degrees:
$ 1.4 \cdot \red{\frac{180^{\circ}}{\pi} } \\ =\frac{252 ^{\circ} }{\pi} \\ \approx 80.2^{\circ} $
So what's the deal with '$$\pi $$ radians' vs 'radians'?
Let's rephrase the question as follows:
Is there any difference between 5 radians and $$ 5\pi \text{ radians }$$?.
Well, let's figure out the answer by converting 5 radians to degrees and $$ 5\pi \text{ radians }$$ to degrees.
If we end up with the same number, then 5 radians and $$ 5\pi \text{ radians }$$ are the same.
$ 5 \cdot \red{\frac{180^{\circ}}{\pi} } \\ =\frac{900 ^{\circ} }{\pi} \\ \approx 286.4^{\circ} $
$$ 5\pi \text{ radians} $$
$ 5\pi \cdot \red{\frac{180^{\circ}}{\pi} } \\ =\frac{900 \pi ^{\circ} }{\pi} \\ =\frac{900 \cancel{ \color{Red} \pi} ^{\circ} }{ \cancel{ \color{Red} \pi} } \\ = 900^{\circ} $
Yes, there is a difference. Remember that $$\pi $$ is a number (approximately 3.1415) so the difference between 5 and 5$$\pi$$ is obvious.
There is no difference, when we put the word 'radian' in the phrase.
$$ 5 \pi \text{ radians} $$ is $$\color{Red}{\pi} $$ times larger than $$ 5 \text{ radians} $$.
ie $$ 5 \pi \text{ radians} $$ is $$\color{Red}{3.1415...}$$ times larger than $$ 5 \text{ radians} $$.
Practice Converting Degrees between Radians
Problem 1
What is the radian measure of 60°?

Use the formula to convert degrees to radians and multiply the degrees by $$ \frac{\pi}{180}$$.
Problem 2
What is the degree measure of an arc whose measure is $$ \frac{2\pi}{3} \text{ radians} $$?

Use the formula to convert radian to degrees and multiply the radian measure by $$ \frac{180}{\pi}$$.
Problem 3
What is the degree measure of an arc whose measure is $$ \frac{7\pi}{12} \text{ radians} $$?
Use the formula to convert radian to degrees and multiply the radian measure by $$ \frac{180}{\pi}$$.
$ \frac{7\pi}{12} \cdot \frac{180 ^{\circ} }{\pi} \\ = \frac{7\pi \cdot180 ^{\circ} }{12\pi} \\ = \frac{7 \cancel{\color{Red}{\pi}} \cdot180 ^{\circ} }{12\cancel{\color{Red}{\pi}} } \\ = \frac{1260 ^{\circ} }{12} = 105 ^{\circ} $
Problem 4
What is the degree measure of an angle whose measure is 14 radians?
Use the formula to convert radian to degrees and multiply the radian measure by $$ \frac{180}{\pi}$$.
$ 14 \cdot \frac{180 ^{\circ} }{\pi} \\ = \frac{ 14 \cdot180 ^{\circ} }{\pi} \\ = \frac{ 2520 ^{\circ} }{\pi} \\ \approx 802.1 ^{\circ} $
How to Convert Degrees to Radians in Pi
Source: https://www.mathwarehouse.com/trigonometry/radians/convert-degee-to-radians.php